(notes by Roberto Bigoni)
Given in a plane Π a straight line d and a point F distant f from d (f > 0), a conic section (or, shortly, a conic) γ is the locus of the points P of Π such that, said H their orthogonal projection on d, the ratio PF/PH has constant value e (e>0).
![]()
The straight line d is the directrix of γ and the point F is its focus.
The ratio e is the eccentricity of γ, f is its focal distance and the product l=ef is its semilatus rectum.
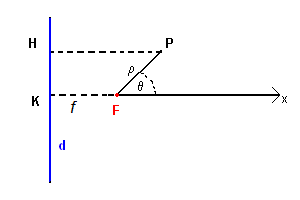
In order to analytically describe a conic section with eccentricity e and parameter f, we can take in Π a polar reference system with pole in F and polar semiaxis given by a ray from F, perpendicular to d and outward oriented with respect to d.
Within this system every point P is in bijective correspondence with a pair of real numbers (ρ;θ), respectively called radial coordinate and angular coordinate of P, such that
where X is a point of the polar semiaxis and the angle θ is counterclockwise oriented, measured in radians and ≤ 2π.
In this reference system the (1.1) becomes
![]()
![]()
The (2.3) is the polar equation of a conic with semilatus rectum l and eccentricity e.
Since the cosine is an even function, that is it has the same value for opposite arguments, the (2.3) shows an important attribute of all the conics: they are symmetrical with respect to the polar axis.
Moreover, from (2.3) we can deduce that, when θ is a right angle, ρ coincides with l;
so l can be geometrically interpreted as the distance of F from the points of γ with polar angles respectively
 .
.
In order to obtain the equation of a conic in cartesian coordinates, we can take a cartesian orthogonal reference system xFy with origin in the focus F, with abscissa axis coincident with the polar axis and with ordinate axis from F.
In this system we have
From the first equation in (3.1) we have
![]()
and, by squaring both sides of the equations in (3.1),

The substitution of the (3.2) into the (3.3) gives
![]()
It must be observed that the (3.4), is more general than (3.1), so the locus given by the latter is a subset of that given by the former one. The name of conic is commonly extended to the loci given by the (3.4).
The (3.4) can be rewritten as
![]()
It can be demonstrated that, if P(xP;yP) is a point on the conic, then the equation of the tangent to the conic at P has equation
![]()
This equation can be authomatically deduced from that of the conic:
If the eccentricity e=0, the (2.3) simply becomes
![]()
that is the conic is the locus of the points with constant distance from the pole F. The conic therefore is the circumference with center F and radius l.
The equation of a circumference in a cartesian plane comes from the (3.4) for e=0
![]()
In a Cartesian frame system XΩY, with axes X and Y parallel to those of the former system and origin Ω(-α;-β), we have
![]()
In this system the coordinates of the center are α e β and the equation (5.2) becomes
![]()
In general, every equation like the (5.3) is the Cartesian equation of a circle with center C(α;β) and radius l.
If we write the variables as x and y, the equation (5.3) becomes
![]()
If we expand the squares of binomials, we obtain
![]()
![]()
If we introduce the parameters
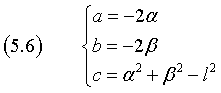
the equation (5.5) becomes
![]()
so the equations like (5.7) represents circumferences with center C(α;β)
![]()
and radius r
![]()
The circumference is real only if the radicand a2+b2-4c is positive.
Circumference through 3 points.
If e=1, the conic is the locus of the points equidistant from F and d and, adopting the word first proposed by Apollonius of Perga, is named parabola.
In this case p coincides with f and from the (2.3) we have
![]()
Since the denominator in the right hand side cannot be null, it follows that the points of a parabola can not have polar angles like 0 or 2π and that when the polar angle approaches these values the polar radius goes to the infinity.
The polar radius has a minimum when the polar angle is π; in this case the polar radius is half of f and the corresponding point V, called the vertex of the parabola, is on the polar axis at equal distances from F an K.
The equation of a parabola in the orthogonal cartesian system xFy follows immediately from the (3.4) with e=1:
![]()
In order to obtain the equation of the same curve in a reference system with the same x-axis and with origin in V, we must apply to the (6.2) the translation
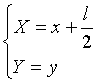
which gives
![]()
From the (6.3) the expression of x in terms of y is
![]()
In general we shall say that every equation
![]()
represents a parabola symmetrical with respect to the x-axis, with vertex at the origin and focus and directrix given by
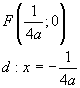
Comparing the (6.4) with the (6.5) we have
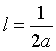
This identity allows you to immediately obtain the (6.5) in polar coordinates.
If, as we did with the circumference, we assume a reference system XΩY, with axes parallel to those of the previous system and with origin Ω(-α;-β), the relations between the coordinates of the two systems are given by
![]()
In XΩY the coordinates of the vertex are α e β and the equation (6.5) becomes
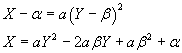
therefore, each equation with the form
![]()
is the equation of a parabola with the axis of symmetry parallel to the abscissa axis and vertex V(α ; β) such that
![]()
that is
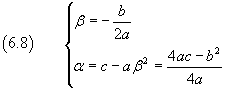
Analogously, every equation
![]()
represents a parabola symmetrical with respect to the y-axis, with vertex at the origin and focus and directrix given by
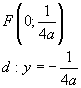
and every equation with form
![]()
is the equation of a parabola with the axis of symmetry parallel to the y-axis and vertex V(α ; β) such that

See also: Area of a parabolic segment
See also: An optical property of the focus
If 0 <e <1, the conic is named ellipse.
In this case, the denominator in the right hand side of the (2.3) is always positive, so every point of the ellipse has finite distance from the focus.
In particular:
the point V' nearest to the focus is that which has polar angle θ=π, for which the distance is
![]()
the point V'' farthest from the focus is that which has polar angle θ=0, for which the distance is
![]()
These two points are the vertices of the ellipse; they, like F, lay on the polar axis.
In the reference system xFy their abscissas results from the (3.4) with y=0
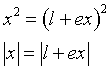

The segment V''V' is called the major axis of the ellipse; its length is
![]()
Let a be the half of this measure:
![]()
Let c be the abscissa of the middle point of V''V':
![]()
From the (7.3)
![]()
and from the (7.4) and the (7.2)
![]()
We can deduce an alternative cartesian equation of the ellipse if we put the origin of the cartesian reference system in the middle point of V''V'.
The equations of the translation are
![]()
Using these equations, from the (3.4) we obtain
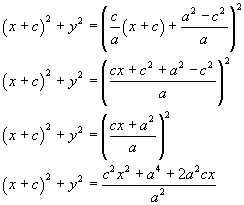
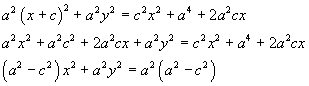
By setting
![]()
we have
![]()
and finally
![]()
The (7.6) is the canonical equation of the ellipse and the reference system is its canonical system.
Since in this equation there are only the squares of the variables, the curve must be symmetrical with respect to both the axes and to the origin. For x=0 we have y = ± b. The parameter b is the measure of the minor semiaxis.
Furthermore, the symmetry of the curve implies that it has another focus beyond that used as pole in the (2.3), which in the canonical system has abscissa -c. So the ellipse has two foci with abscissas ±c.
The equation (7.6) implies a>b. Otherwise, if a<b, the equation represents an ellipse in which the focal axis coincides with the y-axis and the distances |c| of the foci from the origin at the center of the ellipse are given by
![]()
From the (7.6) and the (7.5) we get
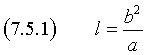
This identity, together with the (7.4), allows you to immediately obtain the (7.7) in polar coordinates.
If we assume a reference system with origin at Ω(-α;-β) and axes parallel to those of the canonical system, the equation of the ellipse becomes
![]()
A conic with eccentricity e >1 is named hyperbola.
Since ρ is positive by definition, also the denominator in the right hand side of the (2.3) must be positive, so the polar angles of the points of a hyperbola are such that
![]()
that is
![]()
The more θ approaches the extremes of this interval, the more ρ increases going to the infinity. Hyperbolas, like parabolas but unlike ellipses and circumferences, are open curves.
The point V' with polar angle θ=π is the nearest to the focus
![]()
V' is the vertex of the hyperbola. But if we let y=0 in the (3.4), we obtain the (7.1). In order to make evident that, if e>1, the two abscissa are both negative, the (7.1) may be rewritten as
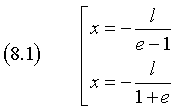
Let V'' be the point with lesser abscissa (the secondary vertex). The segment V''V' is called the real axis of the hyperbola. Its length is
![]()
Let a be the half of this distance. We can write
![]()
As we have seen for the ellipse, it is possible to deduce a cartesian equation alternative to the (3.4) by translating the origin of the reference system from F to O, the middle point of the real axis. In the original system xxFy the abscissa of O is
![]()
therefore
![]()
With the (8.4) from the (8.2) we obtains
![]()
The equations of the translation to the new reference system are
![]()
that, applied to the (3.4), give

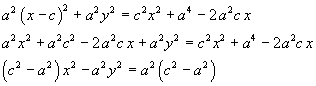
With
![]()
we have
![]()
and finally
![]()
The (8.6) is the canonical equation of the hyperbola and the reference system is its canonical system.
Since in this equation there are only the squares of the variables, the curve must be symmetrical with respect to both the axes and to the origin. Because of this symmetry, the curve has another focus. The two foci in the canonical system have abscissas ±c.
If we rewrite the (8.6) as
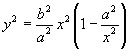
we can see that the curve doesn't have real points in the interval (-a<x<a), so it is formed by two separated branches.
From the same equation we can deduce that the more the absolute value of x increases, the more the equation approaches the following
![]()
which represents a pair of straight lines passing through the origin and having slopes
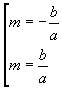
These lines are called the asymptotes of the hyperbola.
The equation (8.7) implies that the real axis of the hyperbola coincides with the axis of abscissas. Equations of form
![]()
represent hyperbolas with focuses on the ordinate axis.
From the (8.6) and the (8.5) we get
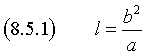
This identity, together with the (8.4), allows you to immediately obtain the (8.7) in polar coordinates.
See also:
Construction of the hyperbola, given the semiaxes a e b.
The area of the hyperbolic segment.
Let d be the equation of the directrix of a conic in the Cartesian plane Oxy
![]()
and Φ(xΦ,yΦ) its focus; the set of the points P(x,y) belonging to the conic with eccentricity e, from (1.1), is expressed by the equation
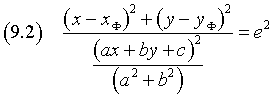
In (9.1) the parameters a, b, c are defined up to a proportionality factor and a and b can not be both null, therefore, without loss of generality, in (9.2) we can assume
![]() so
so
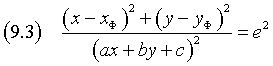
Then
![]()
![]()
![]()
Therefore an equation like
![]()
where the parameters A, B, C, D, E, F are defined up to a proportionality factor k (k≠0), may represent a conic with
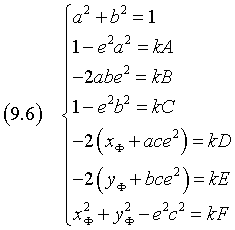
We can see that
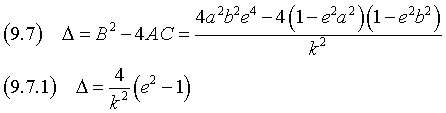
so:
Example.
![]()
Δ = 0: this is the equation of a parabola, so e = 1.
The equation is symmetrical with respect to the bisector of the first quadrant; the focus is on the axis of symmetry, so xΦ=yΦ.
The directrix is perpendicular to the symmetry axis, hence a=b.
From (9.6) we have
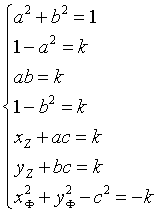
From the first equation we have a2=1/2; b2=1/2
From the third one k=1/2;
From the fifth one
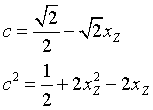
and, finally, from the seventh one, xΦ=0 and c2=1/2. So
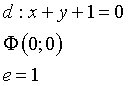

Example.
![]()
Δ < 0: this is the equation of an ellipse.
The equation is symmetrical with respect to the bisector of the first quadrant; the focus is on the axis of symmetry, then xΦ=yΦ.
The directrix is perpendicular to the symmetry axis, hence a=b.
From (9.6), with e≠0 because there is a monomial xy,

From the first equation we have a2=1/2; b2=1/2
Form the third one
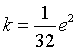
From the second one

From the fifth one

From the seventh one

With  we have
we have
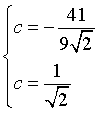
With 

The solutions are equivalent. Then
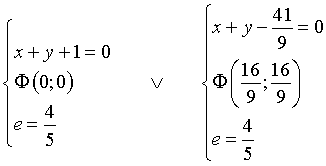
Equation of a conic through five points
General equation of a conic: calculation of the parameters
last revision: May 2025